
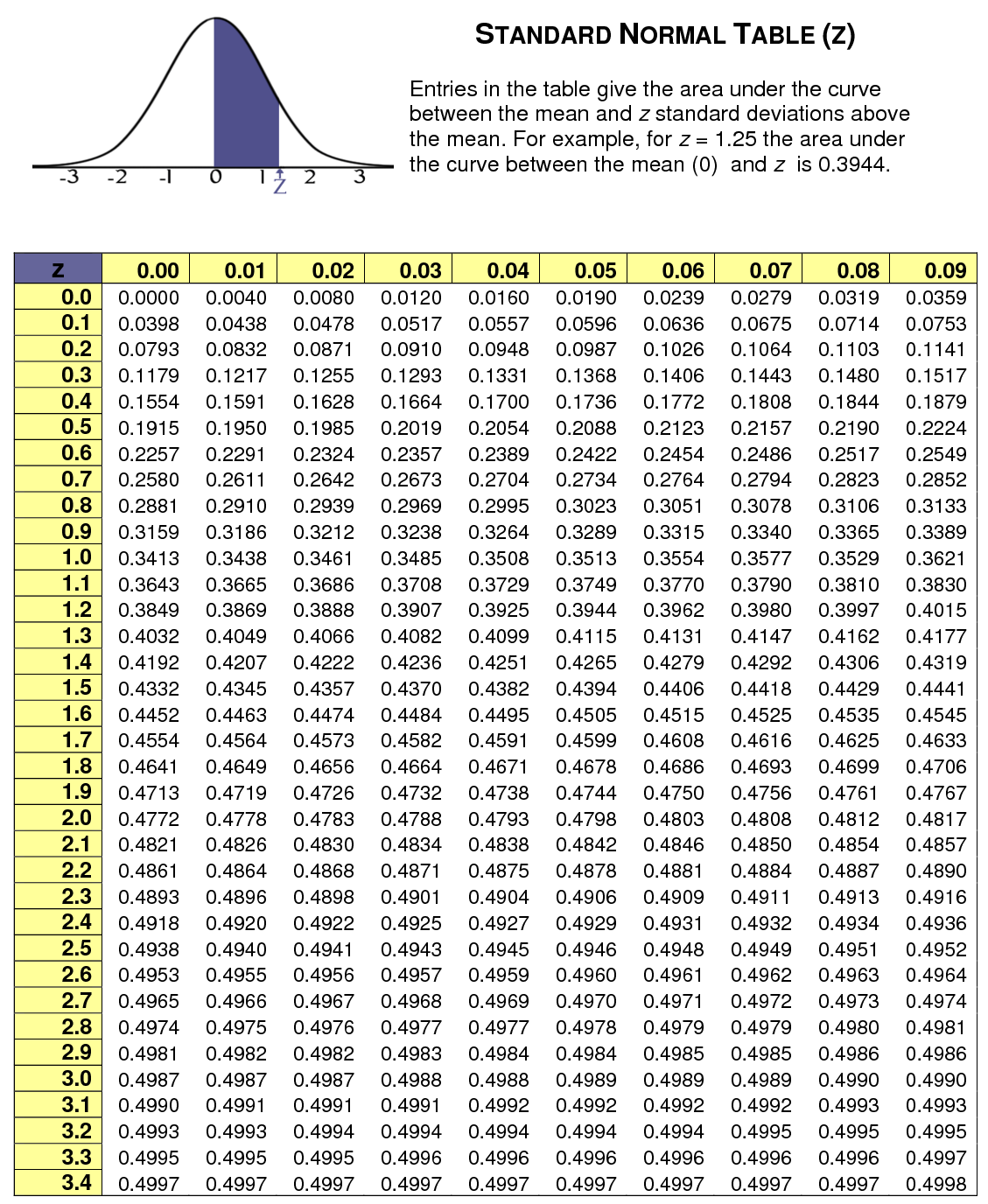
We now go to the standard normal distribution table to look up P(Z>1) and for Z=1.00 we find that P(Z1)=1-0.8413=0.1587. The units place and the first decimal place are shown in the left hand column, and the second decimal place is displayed across the top row.īut let's get back to the question about the probability that the BMI is less than 30, i.e., P(X 35)? Again we standardize: Note also that the table shows probabilities to two decimal places of Z. In this case, because the mean is zero and the standard deviation is 1, the Z value is the number of standard deviation units away from the mean, and the area is the probability of observing a value less than that particular Z value. This table is organized to provide the area under the curve to the left of or less of a specified value or "Z value". Probabilities of the Standard Normal Distribution Z So, the 50% below the mean plus the 34% above the mean gives us 84%. That is because one standard deviation above and below the mean encompasses about 68% of the area, so one standard deviation above the mean represents half of that of 34%.
The table in the frame below shows the probabilities for the standard normal distribution. Examine the table and note that a "Z" score of 0.0 lists a probability of 0.50 or 50%, and a "Z" score of 1, meaning one standard deviation above the mean, lists a probability of 0.8413 or 84%. For any given Z-score we can compute the area under the curve to the left of that Z-score. Since the area under the standard curve = 1, we can begin to more precisely define the probabilities of specific observation. After standarization, the BMI=30 discussed on the previous page is shown below lying 0.16667 units above the mean of 0 on the standard normal distribution on the right. However, when using a standard normal distribution, we will use "Z" to refer to a variable in the context of a standard normal distribution. To this point, we have been using "X" to denote the variable of interest (e.g., X=BMI, X=height, X=weight). For the standard normal distribution, 68% of the observations lie within 1 standard deviation of the mean 95% lie within two standard deviation of the mean and 99.9% lie within 3 standard deviations of the mean. The standard normal distribution is centered at zero and the degree to which a given measurement deviates from the mean is given by the standard deviation. The standard normal distribution is a normal distribution with a mean of zero and standard deviation of 1.


 0 kommentar(er)
0 kommentar(er)
